Tutorials: Electronics fundamentals (ELEFU)
Capacitor C and Inductor L
last updated: 2022-01-30
Quick links
Introduction
Song of this chapter: "The capacitor song" (teacherhaggis on youtube (there's also an inductor song :))
The three elementary linear electronic components are the resistor R, the Capacitor C and Inductor L. Circuits with only linear electronic components are called linear circuits. Their characteristic is that for a sinusoidal input voltage of frequency f, any output of the circuit is also sinusoidal with frequency f. Linear circuits are easier to understand and analyze (they obey the superposition principle) than non-linear circuits.
Important nonlinear electronic components are the diode and the transistor. They will be treated in the next chapter.
The capacitor uses an electric field and the inductor a magnetic field.
Capacitor (condenser) (wiki)
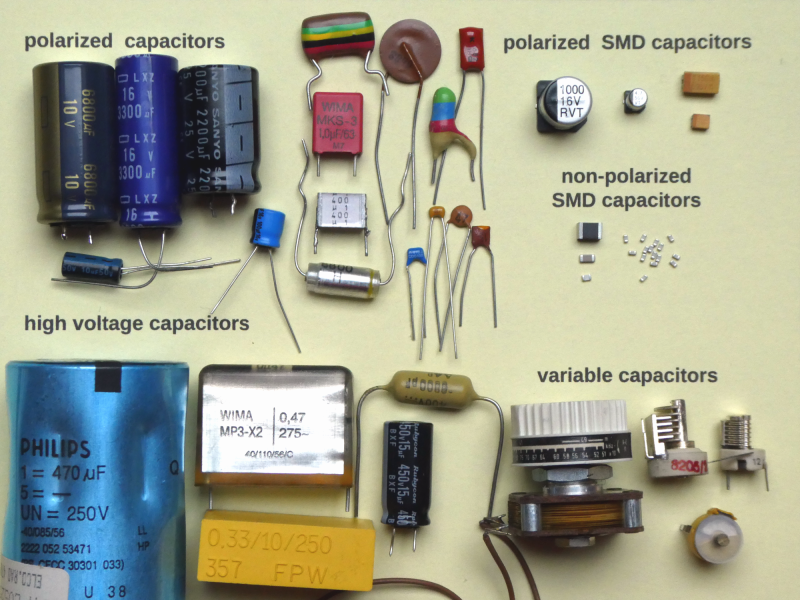
A capacitor is a passive two-terminal component storing electrical energy in an electric field.
Physically, a capacitor is made of two conductors separated by an insulator referred to as a dielectric. This can also be seen in his symbol.
Symbol of a capacitor, a polarized capacitor and a variable capacitor:
For polarized capacitors, the American symbol has a curved plate for the negative side.
"Just do it" C1:
- Build a circuit with an LED (including series resistor) in parallel with a capacitor of 4700 µF (16 V) and in parallel to a voltmeter. The capacitor is polarized! The negative side is marked on the housing. Connect the circuit in series with a switch to a voltage source of 5 V. Draw the circuit by hand.
- Switch the voltage source on and off and describe what happens.
Capacitance C (wiki)
As a resistor has a resistance, the capacitor has a capacitance C (C is used as symbol for the capacitance and also as name for a capacitor). Capacitance is the ability to store energy in the form of an electric field. Capacitance stores charge (electrons).
You know the effect of a capacitor from real world. When you touch the door of a car, you get a slight electric shock. You car is a capacitor. One conductor is the car itself, the tires are the insulator and earth is the second conductor. By friction the car can be charged (acquire a surplus of electrons) to some thousand volt, and when you (a resistance ;)) touch the car a minimal current can flow.
Capacitance exists everywhere, but is mostly very small. The capacitor is a component designed to add specific capacitance to a circuit and can have very high values.
A parallel-plate capacitor, consisting of two conductive plates insulated from each other is a good model to analyse the capacitance. One can conclude by reasoning and then verify in experiments that the capacitance is nearly proportional to the surface area of the conductor plates A and inversely proportional to the separation distance between the plates d.
A third factor is the influence of the material between the plates. This influence is reflected by the permittivity ɛ = ɛ0·ɛr of the insulating (dielectric) material. A part of the permittivity is the relative permittivity ɛr, a material property. ɛ0 is the vacuum permittivity an baseline physical constant:
The permittivity consists of the vacuum permittivity multiplied with the relative permittivity:
The capacitance of a parallel plated capacitor can be calculated with :
The symbol for the capacitance is C (not to confound with the unit of the charge Q, the Coulomb C), the SI derived unit is the farad F.
A capacitor of 1 farad, if charged with 1 coulomb of electrical charge, has a potential difference of 1 volt between its plates.
This gives us another formula for the capacitance:
If we want to calculate the charge Q:
"Just do it" C2:
- Calculate the capacitance of a parallel-plate capacitor with plates from 1 m² and a distance from 1 mm. The dielectric is air.
- Calculate the same capacitance if the dielectric is Mylar (produced in Contern/Luxembourg), and the distance can be reduced to 12 µm.
- Calculate how many electrons can be stored in the Mylar capacitor (U = 5 V).
- Calculate the time needed to discharge the capacitor if you connect an LED drawing 2 mA.
Newer multimeter often have the possibility to measure the capacitance.
"Just do it" C3:
- Read the chapter about measuring a capacitance in the manual of your multimeter. Document the the ranges and the accuracy of this capacitance meter.
- Measure the capacitance of 3 different capacitors and note the imprinted values and the measured values.
Breakdown voltage and temperature (wiki)
The voltage marked on a capacitor is the breakdown voltage of the used dielectric. The breakdown voltage is the maximum voltage that can be applied to an insulator before it conducts. When a current flows through an insulator this is called an electrical breakdown.
Even in (near) vacuum we get a breakdown voltage (vacuum arc). The breakdown voltage of air is about 3 kV/mm ; for Mylar its value is about 430 kV/mm. so the thinner the insulator, the lesser the voltage that can be applied to the capacitor. Normally one uses a capacitor with a marked voltage that is clearly higher than the marked voltage.
Polarized capacitors have a liquid inside and are aging quicker at high temperature. The maximum temperature is marked on the capacitor. For hotter environments it's better to use capacitors with 105° instead of the standard 85°.
Dangers when working with capacitors
A breakdown in a (mostly polarized) capacitor can generate heat that causes the liquid to evaporate very quickly. As the capacitor is sealed, it can turn into an explosive. This is especially true for older capacitors. New capacitors have a special designed top that allows them to vent instead of bursting violently. Defective capacitors have a swollen top.
Capacitors may retain a charge long after power is removed from a circuit!! This charge can cause fatal shocks. When you open a seemingly innocuous camera with flash unit, the flash unit has a capacitor which may contain over 15J of energy and be charged to over 300 volts!! So always discharge capacitors before working on a circuit.
Charging and discharging
Charging a capacitor means accumulating a charge on its conductors. The formula Q = C·U shows that U~Q. The voltage is increasing while charging and we are moving charge (electrons) onto one of the capacitors conductors. This is a current, and we can control with the help of a resistor how quickly the charge moves to the capacitor’s conductor.
Here is the typical circuit to charge and discharge a capacitor:
The capacitor charges through the resistor (switch to power supply (1)). When it the switch connects to ground, the capacitor discharges through the resistor.
"Just do it" C4:
- Build the circuit on your breadboard and charge and discharge the capacitor with 5 V by using the switch (you can move the end of a wire instead of using a switch). Change the resistor to 100 Ω. Note your observations and your conclusions.
- We want to see exactly at what rate the voltage gets higher and lower. This can be done with the oscilloscope (oscilloscope instead of the voltmeter). Test it and document the screen.
- In circuits there is no human switching the capacitor :) but we use signals. We replace the power supply and switch with a frequency generator producing a square wave of 50 Hz. The amplitude should change from 0 V to 5 V. This can be done on the signal generator by adding an offset of 2.5 V to the signal. The square wave replaces now our switch. To get a steady image we change the value of the capacitor to 100 nF and the value of the resistor to 10 kΩ. Build and draw the circuit by hand.
- Get a steady image on the oscilloscope of one period (maximum amplitude) and document it. How long does the capacitor need to charge and discharge?
RC time constant (wiki)
The charging and discharging follows an exponential curve. The formulas for charging:
and for discharging:
When using different capacitors and resistors we see that the time of charging and discharging changes.
By multiplying the value of the resistor with the value of the capacitor we get a time!!
Let's look at the units:
This time is called RC time constant, τ (tau). As shown the unit is seconds.
The RC time constant is the time required to charge a capacitor, through a resistor, from 0 V to 63 % of the value of an applied DC voltage, or to discharge the capacitor through the same resistor to 37 % of its initial charge voltage. This can be calculated with the above formulas.
The time of 2τ charges a capacitor to 85%, and 5τ to 100%.
5τ or 5·R·CCharging and discharging diagram
Measuring current with an oscilloscope
Whats about the current? The only way to see a current is to measure the voltage with an oscilloscope on a resistor in the circuit. Fortunately we have already a resistor in our circuit, so we don't need to add a shunt. The ground of the 2 oscilloscope channels are connected together! Also most grounds of oscilloscopes are connected to the housing and to earth of the power cord. The same is true for the ground of the generator. So it is here not possible to use the oscilloscope like a voltmeter in parallel to the resistor, because we will have a short circuit which can be pretty dangerous when measuring in circuits working with mains voltage (230 V).
!! If you use an oscilloscope all grounds have to be connected together !!
"Just do it" C5:
- Connect the oscilloscope as shown in the second image. Measure the voltage (square wave, 5 V/50 Hz) on the resistor by subtracting both potentials (math function on the oscilloscope). Document the measurement (screenshot).
- Calculate the peak current.
- Why does the current get negative?
Combining capacitors
As seen above, the bigger the surface area of the conductors, the higher the capacitance. If we connect capacitors in parallel, the conductors are combined and we are getting a larger effective surface area. The capacitance gets bigger by connecting capacitors in parallel. With the formula of the capacitance we can show, that the total capacitance is equal to the sum of the individual capacitance's.
When we connect capacitors in series, we are effectively combining the thicknesses of their insulators. A bigger thickness results in a lower capacitance!With the formula of the capacitance we can show, that the total capacitance is the reciprocal of the sum of the reciprocals of the individual capacitance's.
In a parallel circuit of capacitors:
- the voltage is the same for all of the elements.
- the total capacitance is equal to the sum of the individual capacitance's: C = C1+C2+C3+....
- the total capacitance is bigger than any of the individual capacitance's.
- for two capacitors with identical values the total capacitance is the double of the value of one capacitor.
In a parallel circuit of capacitors the total capacitance is equal to the sum of the individual capacitance's.
In a series circuit of capacitors:
- the current is the same for all of the elements.
- the total capacitance is the reciprocal of the sum of the reciprocals of the individual capacitance's: C = 1/(1/C1+1/C2+1/C3+...).
- the total capacitance is always less than the value of the smallest capacitor.
- for two capacitors with identical values the total capacitance is the half of the value of one capacitor.
In a series circuit of capacitors capacitance is the reciprocal of the sum of the reciprocals of the individual capacitance's.
"Just do it" C6:
- Calculate the overall capacitance of the following circuit:
Low-pass filter (LPF) (wiki)
The circuit we used to charge and discharge a capacitor (RC in series) is called an RC low-pass filter.
Let's see why.
"Just do it" C7:
- Use the circuit from "Just do it" C5 exercise. Now change the frequency from 50 Hz to higher and lower frequencies in a wide range (up to 10 MHz) and watch the voltage and current on the capacitor. Document 2 screens and note your conclusions.
- Do the same with a sine wave (
5 VSS, no offset) instead of a square wave. Document 2 screens and note your conclusions.
Ideal filters
Filters are named according to the frequency range of signals that passes through them. The ideal amplitude response characteristics of the four basic filter types is shown in the following image:
We distinguish active filters (containing amplifying devices) and passive filters. A passive filter is a combination of two or more of the three basic linear elements resistors, capacitors and inductors. The RC-filter is a passive filter with two passive components. The output signal has a smaller amplitude than its corresponding input signal, so the passive RC-filters attenuate the signal (gain less than one).
Cutoff frequency (wiki)
The goal of our low-pass filter is to get a high attenuation above a specified frequency and little or no attenuation below that frequency. The specified frequency at which the transition occurs is called the cutoff frequency fc.
The cutoff frequency of a low-pass filter is the frequency at which the output power has fallen to one half the input power. This is the case when the output voltage drops to 0.707 (1/√2, 70.7 %) of the input voltage!
When the voltage drops to 70.7 % of it's value on a resistance, Ohm's law says that the current drops also to 70.7 % of its value. This explains the drop to 50 % for the power:
This cutoff frequency is also called the 3 dB point (see half-power point).
To calculate the cutoff frequency of our RC low-pass we meet again the RC time constant τ:
And so we get the formula for the cutoff frequency:
Example for an RC low-pass filter with C = 2.2 µF and R = 22 Ω:
For the frequency in relation to the cutoff frequency a logarithmic scale is used.
Order of a filter
As seen the filter is characterized by its cutoff frequency (attenuation of the input power by half or 3 dB), but also by the rate of frequency rolloff. The order of a filter determines the amount of additional attenuation for frequencies higher than the cutoff frequency.
The filter frequency response is generally represented using a Bode plot. In this plot the gain (Uout/Uin) is represented in decibel giving us a diagram with two logarithmic scales.
- A first-order filter, reduces the signal amplitude by half (power by 4 or 6 dB), every time the frequency doubles. this makes an attenuation of 6 dB/octave or 20 dB/decade.
- A second-order filter can be build by two first-oder filter and so doubles the attenuation. It attenuates high frequencies more steeply with 12 dB/octave or 40 dB/decade.
- A third-order filter attenuates high frequencies with 18 dB/octave or 60 dB/decade and so on.
"Just do it" C8:
- Get the curve Uout/Uin = f(f) of of the RC low-pass filter (C = 100 nF and R = 10 kΩ) by measuring 10 different reasonable points with the oscilloscope (Upeak). Use a sine wave (2 Vss) to do so. One of the points should be 0.707. Use an calc or excel table to draw the diagram with a logarithmic x axis (including sub ticks).
- Calculate the cutoff frequency and add it to the diagram.
- Draw the same diagram with double logarithmic axis. Note your observations.
- Exchange R and C and do the same work as in the three points above. Note always your conclusions.
Applications
The capacitor has the ability to pass an AC voltage but to block DC voltage. This is very useful, especially in the world of analogue and audio electronics. Often 100 nF capacitors are used on the power lines in a circuit to bypass (shunting) high frequency noise to ground (bypass capacitors). Large capacitors on power lines near regulators are used to smooth slower changes, and to deliver high currents for a short time. Capacitors in combination with resistors are often the basis for timing and oscillator circuits (changing time constant). Capacitors in combination with resistors and inductors are used as filters (see above).
"Just do it" C9:
- To repeat and consolidate this chapter, read the article about the capacitance in the HackSpace Magazine (free pdf download) number 10 (page 74-79).
Next we will see a new component, that is very similar but almost the mirror of a capacitor.
Inductor (wiki)
These chapter on inductors is short. Why?
- Because inductors and capacitors are “mirror images” of each other, and so the concepts discussed above for the capacitors are similar for inductors.
- Inductors are less used, especially in electronics circuits, and can often be replaced by capacitors.
- no time :)
An inductor, (coil) is a passive two-terminal component storing electrical energy in an magnetic field when electric current flows through it.
Physically, an inductor is made of an insulated wire wound into a coil around a core.
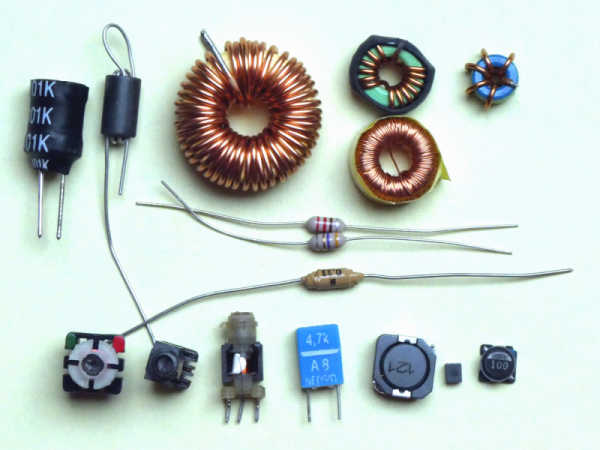
Symbol of an inductor, an inductor with magnetic core and a variable inductor with magnetic core:
Capacitance is the ability to store energy in the form of an electric field.
The inductance L of an inductor is the ability to store energy in the form of a magnetic field.
The symbol for the inductance is L, the SI derived unit is the henri H.
The basic functionality of an inductor is equivalent to that of a capacitor if you swap current and voltage.
"Just do it" L1:
- Read the articles about the inductance in the HackSpace Magazine number 11 (page 78-83).
